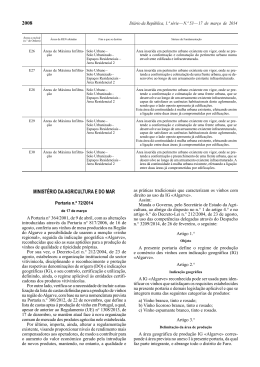
3ª Aula
Modelo Presa- Predador
Formulação, Resolução Numérica,
Potencialidades e limitações.
Modelo Presa-Predador
•Na equação:
dc
 kcn
dt
k  k0 cmax  c  / cmax
Só a logística é que limita o crescimento. Na realidade aparece
sempre um predador que cresce com a presa.
A interacção
entre a presa
e o predador
dá origem a
um sistema
oscilatório.
Modelo de Lotka-Volterra
dPy
dt
 k ny Py  k my Py  G
• Where Py is the concentration of the Prey, Kny is the rate of reproduction of
the Prey and kmy is the rate of natural mortality of the Prey and G is the
grazing rate.
dPr
 E.G  k mr Pr
dt
•
Pr is the concentration of the Predator; kmr is the rate of natural mortality
of the Predator. E is the losing rate (the amount of the Prey destroyed by
the predator, but not used to grow).
g z  Py 
G
Pr
E  Py  k 
•
gz is the grazing rate, representing the amount of food per unit of mass
needed by the predator. k is the semi-saturation constant.
Resolução Numérica
dPy
dt
 k ny Py  k my Py  G
dPr
 E.G  k mr Pr
dt
Pyt dt  Pyt 1  dtkny  kmy   dtG
Prt dt  Prt 1  dtE.G  k mr 
• Nesta discretização admitimos que a produção
e o consumo durante um intervalo de tempo
são função das variáveis no início do intervalo
de tempo: Modelo explícito
Modelo parcialmente implícito


Pyt dt  Pyt 1  dt * kny   dtG / dt1  kmy 


Prt dt  Prt  dt * E * G /1  dt * k mr 
g z t  Py 
G
Pr
P k
E
 y

• Nesta discretização o termo de fonte é
explícito e o termo de poço é implícito.
• O termo de pastoreio (grazing) é explícito para
ter o mesmo valor em ambas as equações.