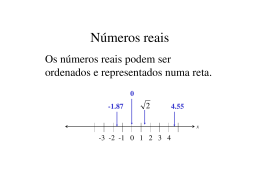
03 - PRODUTOS NOTÁVEIS E EXPRESSÕES ALGÉBRICAS
01) Reduza os termos semelhantes:
a) 3x2  7 x2  8x2  4 x2  7 x2
b) a2b  2ab2  4a2b  2ab2
3x x 2 x x x
   
5 2 5 3 2
d) 8x  4 x2  7 x  3x 2  8x 2
e) 7  x  8x  4  3x  3  5x  2
2a 2 3a 2 3a 2 a 2 4a 2
f)


 
5
2
5
2
5
2
2
2
2
yx
yx 3 yx 5 x y 7 yx 2




g)
3
4
8
12
6
c)
02) Efetue as multiplicações:
a)
 5x y  . 4 xy 
2
2
 3x 2 y 3   2 x3 y 2   xy 2 
 . 
 .

5 
3  4 

b)  
c)
 7 x 2   8ba 2   4 xa3   9b3 

 .
 . 
 .

7  2 
 3  5 
d)
  x  .  2 xy5
e)
f)

2
2

  3y 
 . 
  10 
3a b c  . 4ab 
8a b  . 2a  . ab 
2 3 2
2
3
3
 2a   3b 
 .    .  2ab 
 3  4
g) 
h)

 4 xy z  .  25x
2

2
  5 yx3 
 .

 2 
03) Efetue as divisões:
a)
b)
c)
12a b    4ab 
16m n    2m n 
 20x y    4x y 
2 3
3 2
3
2
2
2
3
 15a3b4c 2   5a 2b3c 4 
 

7

  14 
d)  
 48 x 2 z 4   24 x3 y 
 

15
5 

 
e)  
04) Multiplicação de monômio por polinômio:
a)
 2a b  .3ab
2 3
2
 2a3b2c 2  b4 
b)
 3x  . 2x
c)
2 2 3 2 1 3 3 2
 a  .  ab  a  a 
2
2 
3  4
2
3
 3x 2  2 x  4 
 x2 y3  
6 2
3 3 2
4
d)  
 .  2 xy  x y  x y 
3 
5
2


05) Multiplicação de polinômios:
a)
b)
c)
d)
a
x
2
 4ab2  .  2a3  3ab 
2
 2 x  3 .  2 x  1
1
1 2 3  1
 x  x  . x  
2  3
2
2
 x  2  .  x 2  4 x  .  x 2  2 x  3
06) Efetue as multiplicações:
a)
b)
c)
d)
e)
f)
g)
 3x  . 4x  3x  2
 3x  2 x  .  x  2 x  4 
 4 y  3 y  y  3 . 3 y 
 2x y  . x  4xy  8
 x  2x  3 . 2x  4
 x  x 1 .1  2x 
3
2
2
2
3
2
2
2
2
2
i)  4 x 2  .  4 x3  2 x 2  4 x  2 



k)  x  3x  1 .  2 x  3x  x  3
l)  3x y  2 xy  .   x  2 x 
j)  x 2  x  1 .  x 2  3x  2
2
3
2
2
2
2
2
2
 x  2 . x 1 .  x  2
 2 x 2 x 5   3x 2 6 x 
m) 
   .
 
2 6  4
5 
 3
 3a 2 2a 1   a 2 a 
h) 

  .  
3 2  6 2
 4
07) Divisão de polinômios:
a)
b)
 6x
 4x
2
 13x  6  :  2 x  3
2
 8x3  10  :  2 x  5
08) Determine o quociente das divisões:
a)
b)
c)
d)
e)
 
d)  a  2a  3 : 1  a 
c) x3  1 :  x  2 
 12a b  4ab  8a b  :  4ab 
 2x  x  x  2 :  x 1
 x  5x  4 :  x  4 
 a  2a  3a  :  a  a 
 x  x  24 :  x  3
2 3
3
2
3


f) 10a 4 m3  15a3m2  20a3m3 : 5a 2 m2

2
2
3
2
2
2

h)  x

g) 6a 2  a  1 :  2a  1
4
 2 x3  x 2  6 x  12  :  x 2  3
3
09) O grau do quociente é igual a diferença entre o grau do dividendo e o grau do divisor.
a) Determine o resto da divisão de x4  2 x3  x2  5x  7 por x 2  2 .
b) Determine o polinômio P(x) que multiplicado por x 2  x  1 obtém-se para
produto x3  1 .
c) Determine o valor numérico do resto da divisão de 3x4  5x3  11x  10 por
x 2  2 , quando x  4 .
10) Desenvolva os produtos notáveis:
a)
 x  1
l)  2  x 
b)

c)
 x  y  . x  y 
2
2a

2
e)
 a  2 . a  2 
 a  b  . a  b 
f)
a
d)
2
2
 b  . a  b
3
 ab 2 1 
g) 
 
2
 3
2
3

m)
 m n  m
2
2
 2a 2 3b 
n) 
 
5 
 3
2
2
 3x
  3x

h) 
 y3  .  y3 
 4
 4

i)
 3a  2 xy 
j)
 m2 n3 n 2 m   mn 2 n3m2 



 .

3  3
2 
 2
2
 2 3 a2 3 a 

k) 

 3

2


2
 x 3 4x2 
o) 
 2  3 


2
p)
 2a  3b  . 3b  2a 
q)

5
a3b 4  ab 2
2 6 3 3
r) 
 3  2 



2
2
11) Fatoração de expressões algébricas
A) Fator comum
Exemplos:
a) 5a 2b  15ab2 =
b) 9 x3 y 2  18x 2 y 2  6 x 4 y 
c) 12a3b2  18a2b3  6a3  24a 2b 
B) Agrupamento: aplica-se a polinômios com, normalmente, quatro termos, como
tipo ax  ay  bx  by onde não há fator comum aos quatro termos, porém:
a é fator comum aos dois primeiros termos; b é fator comum aos dois últimos
termos. Então, ax  ay  bx  by = a  x  y   b  x  y    x  y  .  a  b 
Exemplos:
a) 3a  b2  3b  ab 
b) 4ab  2a  2b 1 
x3  x 2  x  1 
d) x4  x3  x2  x 
c)
C) Diferença de dois quadrados: aplica-se a polinômios com dois termos. Temos que
 a  b  . a  b   a2  b2 então a2  b2   a  b  . a  b  bastando extrair a raiz
quadrada de cada um dos termos para encontrarmos a e b.
Exemplos:
x2  9 
a)
x4 1 
c) 9a 2  4b2 
b)
a4 4
d)
 
b2 9
D) Trinômio quadrado perfeito: aplica-se a polinômios com três termos.
 a  b   a 2  2ab  b2 
 trinômios quadrados perfeitos , então:
2
2
2
 a  b   a  2ab  b 
2
Vimos que


a  2ab  b   a  b 
 
2
2
2
a2
b2
Exemplos:
x2  12 x  36 
b) 16a 2  8a  1 
a)
c)
25x2  4 y 2  20 xy 
d)
x2 x 1
  
4 3 9
E) Trinômios do 2º grau: são polinômios com três termos que não são quadrados
perfeitos.
2
2
Observe:  x  a  .  x  b   x  ax  bx  a.b  x   a  b  x  a.b
S  a  b
 P  a.b
Esta última expressão é do tipo x 2  Sx  P, onde 
2
2
Exemplo: x  5x  6  x  (2  3) x  2.3   x  2  .  x  3
Fatorar:
a)
x 2  3x  2 
b)
c)
x2  5x  6 
y2  6 y  8 
d)
x2  x  6 
e) a 2  a  20 
f) 3x 2  6 x  24 
12) Fatore os polinômios: (Para fatorar, inicialmente verificar se o polinômio possui fator
comum. Após, verificar quantos termos tem e em que caso se adapta.)
a)
4 x 2  4 xy  y 2
n) 6a 2 x2  4a 4 x2  12a 2 x 4
b) a 4  4b2
c)
o) 9m2 n4  12m3n3  4m4n2
p) x 2 y 2  2 xyz  z 2
2 x  2 x2  x 1
d) 9a2  30abc3  25b2c6
q) 0, 25  x  x 2
e) 21x3 y3  6 x3 y 4  9 x 2 y 4
r) x3  x 2  x  1
f) 36  12a 2b3  a 4b6
g) xy  x  y  1
s) x 2  5x  6
h)
y 2  16 y  15
i)
x4 1
j)
a 2  2a  35
k)
28a b c  12a b c  4a b c
l)
81x 2  16 y 4
5 4
t) 36  12a 2b3  a 4b6
2 5 2
6 3 3
9 y2
u) 4 x  3xy 
16
2
v) x  7 x  8
2
m) 6 x2  4 xy 2  6 x3 y  8xy
13) Mínimo múltiplo comum de polinômios
Exemplos:
a) Determine o mmc dos monômios 20a 2b3 , 36ab4c2 e 30a3b2 .
b) Calcule o mmc dos polinômios 10a 2 , 5ab  10a, b2  4b  4, ab  2a.
c) Determine o mmc dos polinômios x2  2 x  1, x3  x, 2 x  2.
14) Determine o mmc dos polinômios:
a) 4a 2b3c 4 , 6a3b4c 2
b) 12a3b2 , 18ab3c 2 , 6a 2b2c3
c) 10 x2 y3 z, 15x3 z 2 , 20 y 4
d) 5 p 2 q3 , 15 p3q 2 , 6q 2 , 10 p 4 q
e) m2  n2 , m2  2mn  n2
f) a 2b  ab2 , a 2  2ab  b2 , a 2b4  ab5
g) x2  1, x3  2 x 2  x, x3  x 2  2 x  2
15) Simplificação de frações algébricas: divide-se numerador e denominador da fração
pelo fator comum.
Exemplos:
12a 2b3

18a 3b 2
36m2 n 2

b)
24m3n
2a
c)

ab  2a
x2  2 x  1

d)
x2 1
a)
e)
ax  3a  bx  3b

x 2  7 x  12
16) Simplifique as frações algébricas:
a)
4 a 3b 2
8a 2 b 3
b)
60 x 2 y 3
45 x 4 y 3
c)
x2  4 x  4
x2  4
d)
x4  y 4
x2  y 2
e)
x2  y2
x 2  xy
f)
x3  x 2  x  1
x2  2 x  1
l)
g)
x3  x 2 y
x 2  xy
3a 3b  3ab
m)
a 2b  ab
h)
2 x2 y  x2 y 2
4 xy  xy 3
n)
x 2  3x  2
x3  2 x 2  x  2
i)
x3  4 x 2  4 x
x3  4 x
o)
x4  2x2  1
x2  2x  1
j)
18a 4b3c3
36a 3b 2c3
k)
a3  a 2  ab2  b2
a 2  ab  a  b
x2  6 x  8
x2  2 x