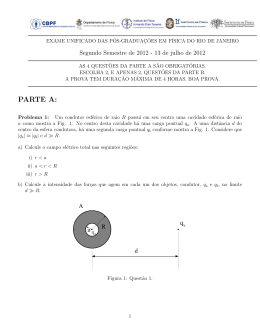
EXAME UNIFICADO DAS PÓS-GRADUAÇÕES EM FÍSICA DO RIO DE JANEIRO
EDITAL 2014-2
Segundo Semestre de 2014 - 06 de maio de 2014
AS 6 QUESTÕES SÃO OBRIGATÓRIAS.
A PROVA TEM DURAÇÃO MÁXIMA DE 4 HORAS. BOA PROVA.
Problema 1: Uma seringa de seção reta A = 1, 0 cm2 colocada na horizontal tem seu êmbolo empurrado com uma força de intensidade F . A área do tubo de escape da seringa é de a = 1, 0 mm2 . Um
lı́quido imcompressı́vel escapa da seringa para a atmosfera com uma velocidade de módulo v = 10, 0
m/s. Consiere o fluxo laminar. Dados: densidade do lı́quido ρ = 1, 0 × 103 kg/m3 , pressão atmosférica
patm = 1, 0 × 105 Pa. Desconsidere atritos.
http://en.wikipedia.org/wiki/Syringe
Figura 1: Problema 1.
a) Calcule a velocidade do êmbolo vE .
b) Calcule a intensidade da força F e a pressão manométrica pM dentro da seringa.
Problema 2: Um pequeno disco de massa m desliza sem atrito sobre uma mesa horizontal, girando
em torno do centro O da mesa. O disco está ligado a um fio ideal, que passa por um orifı́cio no centro
da mesa e suspende na sua outra extremidade um bloco de massa M , inicialmente em repouso. O disco
realiza um movimento circular uniforme de raio R conforme indicado na figura abaixo. A partir de um
certo instante, uma força externa variável puxa o bloco de massa M para baixo. A força externa, então,
se estabiliza e observa-se que o disco passa a girar em torno do centro da mesa, em um movimento
circular uniforme de raio R/2. Considere g a aceleração da gravidade.
1
Figura 2: Problema 2.
a) Qual a velocidade angular do disco no movimento circular inicial de raio R?
b) Qual a nova velocidade angular quando o movimento circular passa a ter raio R/2?
c) Qual o trabalho realizado pela força externa que puxou o fio para que haja a mudança de movimento
circular de raio R para R/2?
Problema 3: Um fio reto e longo transporta uma corrente i e está no mesmo plano de uma espira
quadrada de lado a. A espira está inicialmente a uma distância r0 do fio e se move perpendicularmente
a ele com velocidade constante ~v , como mostra a Figura 3.
Figura 3: Problema 3.
A espira tem resistência R. Determine:
a) O fluxo magnético através da espira na posição indicada na figura.
b) A f.e.m. induzida em função do tempo.
c) A intensidade e o sentido da corrente induzida na espira em função do tempo.
Problema 4: O efeito Compton consiste em um espalhamento relativı́stico onde um fóton de energia E0 e momento p~0 incide contra um elétron livre inicialmente em repouso. Dado que o ângulo de
espalhamento do fóton em relação a sua trajetória incidente é θ, determine:
2
a) As leis de conservação para a energia e momento na colisão.
b) A energia do elétron após a colisão em termos das energias do fóton antes e após o espalhamento.
c) A diferença de frequência do fóton após e antes do espalhamento em termos da massa do elétron e o
ângulo de espalhamento.
Problema 5: Para estados puros, o operador densidade é definido como: ρ = |ψi hψ|, sendo |ψi o
ket que define o estado quântico de um sistema.
Para
|e0i e |e1 i, a matriz
um sistema de 2 nı́veis
ρ11 ρ12
0 1
0 −i
densidade tem dimensão 2 × 2, ou seja: ρ =
. Sabendo que: σx =
, σy =
ρ21 ρ22
1 0
i 0
1 0
e σz =
:
0 −1
a) Mostre que para um estado puro tr (ρ) = 1 e o valor médio de um observável qualquer, A, é dado
por: hAi = tr (ρA). (tr = traço)
√
b) Mostre que ρ12 = ρ∗21 e determine a matriz densidade para o estado |ψi = (|e0 i + i |e1 i) / 2, descrito
na base formada pelos próprios vetores |e0 i e |e1 i.
√
√
c) Ainda para um sistema de 2 nı́veis, mas para outro estado em que hσx i = 1/ 2, hσy i = 1/ 2,
hσz i = 0, determine os elementos da matriz densidade.
Problema 6: Considere o problema unidimensional de uma partı́cula de massa m, sem spin, confinada
em uma região de comprimento L. As paredes de confinamento são intransponı́veis de forma que o
potencial nas extremidades desta região de confinamento deve ser tomado como um potencial infinito.
a) Encontre a função de onda que descreve esta partı́cula. Não é necessário normalizar.
b) Generalize o item (a) de forma a descrever uma partı́cula confinada em uma caixa cúbica de aresta L
cujas faces também são intransponı́veis e encontre a função de onda tri-dimensional desta partı́cula.
Não é necessário normalizar.
c) Encontre as auto-energias da partı́cula na caixa cúbica e calcule a degenerescência do primeiro estado
excitado.
3
EXAME UNIFICADO DAS PÓS-GRADUAÇÕES EM FÍSICA DO RIO DE JANEIRO
EDITAL 2014-2
Second Semester 2014 - May 6th , 2014
YOU MUST SOLVE ALL 6 PROBLEMS.
YOU CAN USE UP TO 4 HOURS TO SOLVE THE EXAM. HAVE A GOOD EXAM.
Problem 1: The plunger of a syringe, laid out horizontally, with internal horizontal cross section
A = 1, 0 cm2 is pushed in with a force of intensity F . An incompressible liquid escapes to the atmosphere
through a hole with a cross section a = 1, 0 mm2 , with speed v = 10, 0 m/s. Consider the flow to be
laminar. Data: liquid density ρ = 1, 0 × 103 kg/m3 , atmospheric pressure patm = 1, 0 × 105 Pa. Neglect
any friction process.
http://en.wikipedia.org/wiki/Syringe
Figure 1: Problem 1.
a) Calculate the plunger velocity vE .
b) Obtain the force intensity F and the manometric pression pM inside the syringe.
Problem 2: A small disk of mass m slides without friction on a horizontal table, in uniform circular
motion of radius R around the center O. The disk is connected to an ideal string that passes through
a small hole at O and holds a block of mass M , initially at rest, as shown in the figure. A variable
external force starts pulling the block downwards. At some point the external force stabilizes a new
uniform circular motion with radius R/2. Use g for gravity acceleration.
a) What is the disk’s angular velocity for the circular motion of radius R?
1
Figure 2: Problem 2.
b) What is the disk’s angular velocity for the circular motion of radius R/2?
c) How much work has been done by the external force to change the circular motion from radius R to
R/2?
Problem 3: A straight and infinite wire carries a constant current i. A square loop of side a is located
in the same plane of the wire, at an initial distance r0 . The loop is moving away from the wire with
constant velocity ~v , as indicated in the figure 3.
Figure 3: Problem 3.
Let R be the resistance of the loop. Determine the following:
a) The magnetic flux through the loop at its initial position.
b) The electromotive force (emf) induced on the loop as a function of time.
c) The intensity and direction of the induced current on the loop as a function of time.
Problem 4: The Compton effect consists of a relativistic scattering in which a photon of energy E0
and momentum p~0 collides with an electron initially at rest. Let the photon scattering angle relative to
the incident trajectory be θ. Determine:
a) The collision conservation laws for energy and momentum.
2
b) The energy of the electron after the collision in terms of the energy of the photon before and after
the scattering.
c) The frequency change of the photon after and before the scattering in terms of the electron mass and
the scattering angle.
Problem 5: Pure quantum states can be described by its density operator ρ = |ψi hψ|, where |ψi
is the ket that defines the state of a system. In the case of atwo level system,
i.e. |e0 i and|e1 i, the
ρ11 ρ12
0 1
density matrix has dimension 2 × 2 so that we can write ρ =
. Given that σx =
,
ρ21 ρ22
1 0
0 −i
1 0
σy =
and σz =
:
i 0
0 −1
a) Show that tr (ρ) = 1 and the mean value of an arbitrary observable A is given by hAi = tr (ρA). (tr
= trace)
√
b) Show that ρ12 = ρ∗21 and determine the density matrix for the state |ψi = (|e0 i + i |e1 i) / 2 written
in the vector base expanded by |e0 i and |e1 i.
√
c) Determine√the components of the matrix density for a two level system in the case where hσx i = 1/ 2,
hσy i = 1/ 2, hσz i = 0.
Problem 6: Let a spinless particle of mass m be confined in an onedimensional region of size L.
Consider the confinement walls to be impenetrable so that it can be modeled as infinite potential walls.
a) Find the wave-function of the particle. (Normalization is unnecessary).
b) Generalize item (a) to describe a particle confined in a cubic box of edge L which also has impenetrable
walls. (Normalization is unnecessary).
c) Find the energy eigenvalues of the particle in the cubic box and calculate the degeneracy of the first
excited state.
3