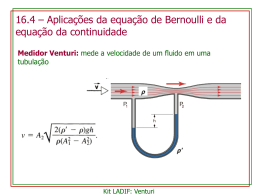
Aula Teórica 12
Equação de Bernoulli
Bernoulli’s Equation
• Let us consider a Stream pipe such as indicated in
the figure and an ideal fluid
(without viscosity) .
• Using the mass and
momentum conservation
principles,
• obtain an equation relating
the energy in two sections.
Mass conservation
• Being a stream pipe there is flow on the tops
only.
Performing a mass balance

dV  m out  m in  0;

t CV

dV  dm  0

t CV

 Ads  dm  0
t
Below we will use:
 

V   Ads  dm   0
 t

Momentum Balance
Forces
 

V   Ads  dm   0
 t

Bernoulli’s Equation
Considerations
• The Mechanical energy remains constant along a
streamline in steady, incompressible, frictionless
flow.
• Pressure is a form of energy: is the energy (work)
necessary for moving a unit of volume from a
region with null pressure into a region of pressure P.
• Total pressure:
• Piezometric Pressure:
Ptotal
1
 P  V 2
2
Ppiezo  P  gz
Problems
Nozzle: compute the force knowing
the discharge.
Chaminé
• Considere uma chaminé que escoa um gás
cuja massa volúmica é 1.1 kgm-3 relacione a
velocidade à saída com a altura da chaminé e
com a massa volúmica do ar exterior.
A equação de Bernoulli só é aplicável se as
propriedades do fluido forem uniformes e
por isso pode ser aplicada no interior da
chaminé ou no exterior, mas não para
relacionar pontos do interior com pontos
do exterior. A diferença de pressões entre
a entrada e a saída da chaminé é
determinada pelas condições exteriores:
Chaminé - Resolução
• Considere o escoamento num tubo de
Ventouri cuja área de entrada (e saída) é de 5
cm2 e na garganta é 2 cm. Se o fluido que
circula no Ventouri for ar e h for 10 cm de
água, determine o caudal que circula no
Ventouri.
h
The Energy Equation
• Let us consider a control volume, and apply
the Reynolds theorem to the Energy
conservation principle.
• If we assume
– uniform properties at the inlet and outlet,
– Steady conditions and incompressible and
adiabatic flow.
• We will obtain a generalised form of the
Bernoulli’s equation.