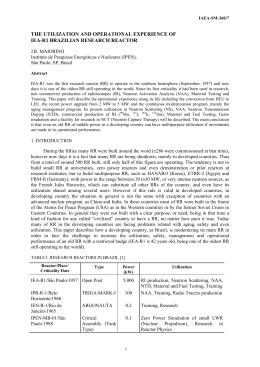
PRELIMINARY INVESTIGATION OF THE GLOBAL KINETIC
PARAMETERS OF LOW-RANK COALS UNDER OXY-FUEL CONDITIONS
Adriano Carotenuto1, Rodrigo Corrêa da Silva2 , René Lúcio Rech1, Nilson Romeu Marcílio1,
Paulo Smith Schneider1, Hans Joachim Krautz2
1
2
Federal University of Rio Grande do Sul
School of Engineering
Rua Sarnento Leite 425, 1° andar
Bairro Cidade Baixa, 90050-170
Porto Alegre – Brazil
Brandenburg University of Technology Cottbus
Chair of Power Plant Technology
Universitätsstrasse 22 (MZG)
03013 Cottbus – Germany
ABSTRACT
The present work is aimed to investigate the global kinetic parameters of low-rank content coals by using an
atmospheric cycloidal combustion reactor. This experimental facility provides the possibility to evaluate the combustion
process under more realistic conditions, i.e. high turbulent and swirling flows, covering a temperature range similar to
those found in practical furnaces. In addition, different mixtures of oxidant can be utilized, such as air and mixtures of
O2 and CO2. The global kinetic parameters are determined with the aid of gas-potentiometric analysis by using oxideion conducting solid electrolytes as stabilized zirconia. Investigations were carried out with two high ash content coals
deriving from mines located in Southern Brazil (Camada Bonito and Mina Leão) and one pre-dried lignite from
Germany. The complete burn-out of the samples were evaluated under air-firing and oxy-fuel conditions (21 vol.% O2
and 79 vol.% CO2) in three different temperature levels (1073, 1173 and 1273 K). In this work, a detailed description of
the experimental method and the preliminary results obtained for the overall combustion time and kinetic parameters
are presented and discussed.
Keywords
Oxy-fuel combustion, global kinetic parameters, high-ash content coals, lignite
1.INTRODUCTION
Among the possible technologies for emissions control from coal-fired power generation, CO2
capture and storage (CCS) has been recognized as a promising option for achieving significant
results, where oxy-fuel technology is pointed as one of major options in the near-future term (Wall,
2007; Olajire, 2010). In this technology, a combination of almost pure oxygen and recycled flue gas
are used for combustion of the fuel instead of air. By recycling the flue gas, a gas consisting mainly
of CO2 and water vapor is generated, almost ready for sequestration without stripping of the CO2
from the gas stream. Additionally, the recycled flue gas is used to control flame temperature and
make up the volume of the missing N2 to ensure there is enough gas to keep an approximated heat
transfer conditions (Toftegaard et al., 2010). The different physical and chemical properties of CO2
when compared to N2, in combination with its higher partial pressure, have motivated several
studies to understand the impacts on combustibility, pollutant emissions and heat transfer. In terms
of combustion, besides the influence on devolatilization and ignition of coal particles, the burnout
of both char and volatiles is expected to be affected (Wall, 2007 and Toftegaard et al., 2010). A
completely understand of these effects is an essential requirement for the determination prediction
of coal combustion by mathematical modeling via Computational Fluid Dynamics (CFD). To assess
the combustion behavior, trials were carried in a 20 KWth experimental facility at the Chair of
Power Plant Technology of the Brandenburg University Technology Cottbus, which utilizes a
cyclone reactor for combusting the fuels. In this work, data is reported for German pre-dried lignite
and two Brazilian high ash content coals, whose information on the combustion process under oxyfuel conditions are still very limited.
2.DESCRIPTION OF THE LABORATORY FACILITY
2.1General overview
A schematic diagram of the experimental setup is shown in Fig. 1. The combustion takes place
in an atmospheric cyclone reactor with a rated capacity of 20 kWth. The geometry of the reactor
seems to be a simplified modification of standard cyclone dust separators to a combustor
configuration, where the fuel is added to the tangential oxidizer stream. Injecting coal and the
oxidizer tangentially into the reactor causes the centrifugal force to throw the particles on the walls
of the combustion chamber.
Figure 1. Schematic of test facility and measurement points (T: temperature, P: pressure, V: gas flow).
Crushed and pre-dried coal is previously transported into a storage silo which feeds a metering
system capable of maintaining a steady flow regime of fuel up to the reactor. The size of the coal
particles for continuously operation is limited first of all by possibility to burn out during the
particle residence time within the combustion chamber and also by the necessity of keeping the
particles in suspension to avoid depositing them on bottom of the furnace. Thus, the particle size
used in this reactor should not be smaller than 50 µm and should not exceed 2,000 µm. The
temperature of coal in the feeding system is approximately 300 K. The coal can be also inserted into
the reactor discontinuously by means of an independent system for investigation of rates of the
combustion process of single samples.
The combustion air is supplied by a fan of variable speed, whereas in the case of oxy-fuel
combustion, O2 and CO2 are supplied by storage tanks. In this case, the gas flow is controlled with
pressure regulators and valves and the flow rate measured using calibrated rotameters. Additionally,
the flow rate upstream of the furnace are measured with an orifice plate. In order to increase the
temperature of oxidant stream before entering into the furnace a preheater and a gas-burner operated
with propane are installed. The oxidizer is injected into the bottom of the reactor with a temperature
around 900 K.
After the reactor, the flue gas is passed through a cyclone dust separator to remove the fly ash
and other particulates before being cooled with an air quench and directed to the stack. The pressure
within the furnace controlled by an induced draft fan is maintained near to the atmospheric pressure
to reduce the contamination from the air in-leakage.
For the calculation of energy and mass balances, values of pressure, temperature, and species
concentrations are continuously registered at several points. Gaseous samples are collected and
conducted to the gas sample preparation and analysis through electrically heated tubes maintained
at 473 K. The gas is cooled and the moisture content is removed before it enters the analyzer.
Therefore, all the standard gaseous concentrations, i.e. O2, CO2, NO, CO and SO2 are measured on
a dry basis. Additionally, the H2O and O2 amount in the oxidizer stream are also monitored.
2.2Cyclone combustion reactor
The combustion chamber shown in Fig. 2, is constructed in stainless steel with a length of 1.1 m
and an inner diameter of 0.140 m. The oxidizer is injected tangentially at the bottom of reactor,
whereas the coal particles are injected perpendicularly to the wall at 0.175 m distant from the
bottom. A three times reduced exit of the reactor compared to this diameter is installed at the top.
The reactor volume where the reaction takes place is 0.0075 m3. Due to its geometry, intensive
rotation of the flame within the reactor caused by high velocities, allows a higher burnout while the
whirling flames moves upward. The ratio of angular momentum to the axial momentum of the flow
(swirling number) is approximately 8.5. This value is strong enough to create an inner recirculation
in the centre of the combustion chamber and able to provide a stabilized flame for wide range of
coals, including those which are normally considered difficult to burn efficiently, such as high ash
content coals, lignites and anthracites. Although the reactor is relatively small, the physical
phenomena of full-scale furnaces are reproduced, i.e. combustion under a fully turbulent flow
combined with significant heat transfer by radiation, which provides high heating rates of a cloud of
particles until a complete burnout. The combustion reactor is electrically heated enabling a constant
wall temperature up to 1350 K.
Temperatures along the inner surface of the reactor are measured using three inconel sheathed
chromel-alumel type ‘K’ thermocouples positioned according to the Fig. 2. On the top of the
reactor, a very simple camera system is utilized to register the image of the combustion. For the
investigation of kinetic parameters, an oxygen-solid electrolyte sensor is also positioned 0.01 m
distant from the inner lateral wall of the reactor in order to acquire the oxygen partial pressure
within the combustion chamber.
Figure 2. Cyclone combustion reactor (measures in meter).
3.EXPERIMENTAL APPROACH TO DETERMINE KINETIC PARAMETERS
3.1Test procedure
The test procedure for obtaining the global kinetic parameters from this test facility was
extensively studied by Tappe (2009). Basically, this test facility is continuously fed with coal in
order to reach high temperature levels in the reactor. When the temperature set in the reactor is
reached, the continuously fuel feeding is stopped in order to increase the oxygen concentration
within the reactor. As consequence, all the three measured points of temperature decrease. When
new steady conditions are established, i.e. constant temperatures and oxygen concentration, the
sample is inserted into the reactor through a separating feeding system.
Fig. 3 illustrates the behavior of the oxygen and temperature within the combustion chamber
during a trial of a 3-g-sample of lignite under air conditions at 1073 K. As can be observed, the
starting point of the combustion sequence is characterized by the sudden drop of the oxygen
concentration due to the combustion of volatile matter right after the injection of the sample,
followed by the combustion of the char mass until the oxygen concentration reaches initial O2
concentration. Afterwards, the continuously feed is restarted in order to reach temperature
conditions to further test.
Determination of global kinetic parameters is based on registered values of oxygen
concentration measured by oxygen-solid electrolyte sensor and time average temperatures measured
by the three thermocouples during the combustion of the sample. As can be observed in the
registered temperature profiles depicted in Fig. 3, temperature gradients are developed within the
reactor, due to the characteristics of the system utilized. The combustion takes place mainly at the
bottom of reactor, where higher temperatures are observed. As the time increases, the coal particles
are dragged upwards within the combustion chamber, where the solid electrolytic oxygen sensor
and the other two thermocouples are located.
Temperature (K)
Restart of
continuously feed
Combustion
of the sample
Stop of
continuously feed
22
1150
20
1100
18
1050
16
Temperature at Bottom
Temperature at Middle
Temperature at Top
O2 (vol. %)
1000
O2 (vol. %)
1200
14
950
12
0
50
100
150
200
Time (s)
Figure 3. Oxygen and temperature curves of a representative test.
3.2 Experimental conditions
In the present study, trials were performed with a German pre-dried lignite (LTBK) from the
Lusatian region and two high-ash content coals derived from Leão and Bonito mines located in
Southern Brazil. The proximate and ultimate analyses of the coals are given in Table 1. The
combustion of the samples, sieved to a size range of 1,000-2,000 µm, was carried out under airfiring and oxy-fuel conditions. The fundamental approach for all experiments conducted was to
keep baseline air-firing tests comparable to those performed under oxy-fuel conditions as similar as
possible. In order to keep similar velocities within the combustion reactor the volumetric flow of the
oxidizer was kept approximately constant for all experimental cases. Additionally, the reactor
temperatures monitored by the K-type thermocouples installed within the furnace were kept
constant at three different levels (1073, 1173 and 1273 K). The reactor operating conditions are
summarized in the Table 2. In order to test the repeatability of the experimental data, experiments
have been carried out with two to three representative replicates, following the procedures of the
design and analysis of experiments proposed by Montgomery (2001).
Table 1. Proximate and ultimate analysis of tested coals.
Proximate analysis (weight %, dry)
Volatile matter
Fixed Carbon
Ash
Ultimate analysis (weight %, daf)
Carbon
Hydrogen
Nitrogen
Sulfur
Oxygen (by difference)
Lignite
Leão
Bonito
54.09
39.39
6.52
19.97
28.96
51.07
12.60
21.98
65.42
67.05
6.95
0.70
0.80
24.50
73.62
5.16
1.31
4.67
15.24
77.61
5.02
1.33
10.52
5.53
Table 2. Experimental parameters for air-firing and oxy-fuel.
Experimental condition
O2 in oxidizer (vol. %)
H2O in oxidizer (vol. %)
Oxidizer volume flow (m³/h)
Oxidizer mass flow (kg/h)
Oxidizer temperature (K)
Reactor temperature (K)
Sample weight (g)
Air-firing
Oxy-fuel
21
2
21.50
7
2.2
23.50
11
923
1073 , 1173 , 1273
1
4.GLOBAL KINETIC FORMULATION
The formulation described in the next sections is based on the oxygen concentration registered
by the solid electrolyte oxygen sensor during the combustion of coal sample as detailed in Fig. 3.
The fundamentals of formulation presented in this study followed the most relevant works carried
out previously by Levenspiel (1999), Lorenz and Rau (1998) and Turns (2006).
4.1Balance of oxygen
In order to apply the law of mass conservation of oxygen, a control volume assuming the reactor
is utilized where
nO2 ,in  nO2 ,out  nO2 ,cons
(1)
The oxygen molar flow at the inlet and outlet of reactor are calculated by multiplying the
concentration by the volumetric flow rates (Lorenz and Rau, 1998). The resulting difference by the
molar flow rates at inlet and outlet is the oxygen molar flow rate consumed by the combustion of a
sample and given by
nO2 , cons  CO2 , in Vin  CO2 , out Vout
(2)
The oxygen molar flow rate consumed during the combustion of a sample can be expressed in
terms of the temperature, oxygen molar fraction and pressure, by following the ideal gas law
relationship according to Eq. (3).
nO2 ,cons 
1000
1000
Patm f O2 ,in Vin 
Patm f O2 ,out Vout
RTin
RTout
(3)
Assuming a volumetric flow rate and temperature within the reactor as reference, Eq. (3)
becomes
nO2 ,cons 

1000
PatmVout f O2 ,in  f O2 ,out
RTout

(4)
4.2Oxygen mass consumption
The amount of oxygen consumed during the combustion reaction of a sample is calculated from
the Eq. (4), by integrating it along combustion time.
N O2 , cons 


1000  t
PVout o f O2 ,in  f O2 , out dt
RTout
(5)
4.3Reaction rate equation
The equation to describe the rate of the oxygen consumed during the combustion of a sample is
expressed in terms of the oxygen conversion and initial concentration of oxygen as
 rO   CO ,0 dXO
2
2
2
dt
(6)
The equation of the conversion is calculated by knowing the moles of oxygen consumed
( NO 2,cons ) until a specific time, divided by total of moles of oxygen consumed until the end of the
combustion, as shown in Eq. (7).
X O2  N O2 , cons N O2 , tot
(7)
4.4Integral method of analysis
The integral method of analysis is utilized to calculate the global kinetic parameters of the
sample combustion, i.e. the order of reaction and the reaction rate constant (Levenspiel, 1999).
Here, the coal reaction rate is approximated by an unimolecular irreversible reaction, according to
Eq. (8).
 rO   keff COn ,0 1  X O  n
2
2
2
(8)
As the combustion of solids is characterized by heterogeneous reactions, an effective reaction
rate constant is introduced in Eq. (8) in order to represent both the phenomena of mass transfer and
chemical reaction kinetics (Turns, 2006).
By combining the Eq. (6) and Eq. (8) and reorganizing the terms before integrating the
following expression is obtained.
 dX O2 (1  X O2 ) n  keff . CO( n ,01) dt
(9)
2
The reaction order and effective constant rate are obtained by starting the order of reaction
between 0 and 1, and followed by the integration of Eq. (9). Afterwards the reaction rate obtained
by linear regression is supposed to be verified in terms of its correlation coefficient (R2), which
must be close to unity. It is worthy to mention that the angular coefficient of the right side of Eq. (9)
determines the value of constant reaction rate, which depends on the initial oxygen concentration.
In order to verify the profile of the effective constant rate for a specific value of reaction order, Eq.
(9) can be rearranged into Eq. (10). Thus, the behavior of constant rate along the time for a known
conversion reaction rate and by keeping a constant reaction order can be verified.
k eff (t )  (CO2 ,0 )1 n (1  X O2 )  n dX O2 dt
(10)
In order to verify the accuracy of the reaction rate calculated with the kinetic parameters by the
integral method, the reaction rate is multiplied by the volume of the reactor to derive the rate of
molar oxygen consumed, and it is compared with the rate of molar oxygen consumed calculated by
the oxygen mass conservation Eq. (4).


V  rO2  nO2 , cons
(11)
4.5Overall activation energy
The overall activation energy calculated for different temperature levels is obtained by the
linearization of the Arrhenius’ law according to Eq. (12), which is a well-known method for the
temperature dependence of reaction rate constant. The values of overall activation comes from the
angular coefficient (E/R) fitted for the temperature range of experimental data. This method has
been strongly suggested from various standpoints as being a good approximation to the true
dependency of both collision and transition-states theories (Levenspiel, 1999).
ln k eff  ln k 0  E / RT
(12)
5.RESULTS AND DISCUSSIONS
The initial temperatures within the reactor before injecting the coal samples were uniform for all
cases with a variation of  10 K, whereas the initial oxygen concentration was maintained with a
variation of  0.1 %. In order to smooth the oxygen concentration due to the fluctuations presented
by the oxygen sensor, a moving average of three registered values was applied. The results obtained
during the experimental tests presented a good repeatability in the values of oxygen concentration
as can be observed in Table 3. Additionally, the standard deviation of the moving average varies
from 0.01 to 0.10 % with average of 0.05%.
Table 3. Average of standard deviations of the oxygen concentration moving average values obtained for all replicates
separated by temperatures, atmospheres and coals.
Lignite coal Leão coal Bonito coal Temperature
(K)
1073
1173
1273
1073
1173
1273
1073
1173
1273
Air (%)
0.10
0.10
0.05
0.11
0.04
0.09
0.03
0.05
0.02
Oxy-fuel (%)
0.10
0.17
0.05
0.03
0.17
0.09
0.02
0.08
0.08
5.1Oxygen concentration curves
The behavior of O2 concentration during the burnout tests of lignite under air and oxy-fuel
conditions for three temperature levels are plotted in Fig. 4. Significant differences were observed
under oxy-fuel atmospheres at 1173 and 1273 K. This O2 consumption at higher temperatures is
22
22
20
20
18
18
O2 (vol. %)
O2 (vol. %)
explained by the gasification reaction due to higher partial pressure of CO2 under oxy-fuel condition
(C + CO2  2CO). This reaction is heavily affected by temperature, increasing its reaction rates
from 1073 K (Rathman, 2009; Stanmore, 1998; Zhang et al., 2010).
16
16
1073 K
1173 K
1273 K
1073 K
1173 K
1273 K
14
14
12
12
0
5
10
15
20
25
0
5
10
time (s)
15
20
25
time (s)
(a)
(b)
Figure 4. Oxygen concentration curves of pre-dried lignite under air-firing (a) and oxy-fuel (b).
22
22
20
20
18
18
O2 (vol. %)
O2 (vol. %)
Similar plots for the other two investigated coals are depicted in Fig. 5 and 6. It becomes evident
that the reactivity of the coals under similar conditions is preliminary determined by the rank, since
the slope of O2 concentration for high-ash content coals is considerably lower than lignite.
Nevertheless, a higher slope is observed when the temperature increases. The results also assessed
the influence of gasification reaction by CO2 under oxy-fuel condition in both high ash content
coals. Significant differences are mainly observed for Leão coal at 1273 K, whereas the Bonito coal,
which has more than 60 weight % of ash, presented a very small influence.
Overall combustion times are longer under oxy-fuel compared to air air-firing. This is explained
by the increasing of the O2 consumption due to the reaction with CO (CO + ½ O2  CO2).
16
16
1073 K
1173 K
1273 K
1073 K
1173 K
1273 K
14
14
12
12
0
5
10
15
time (s)
20
25
0
5
10
15
time (s)
(a)
(b)
Figure 5. Oxygen concentration curves of Leão coal under air-firing (a) and oxy-fuel (b).
20
25
22
20
20
18
18
O2 (vol. %)
O2 (vol. %)
22
16
16
1073 K
1173 K
1273 K
1073 K
1173 K
1273 K
14
14
12
12
0
5
10
15
20
0
25
5
10
15
20
25
time (s)
time (s)
(a)
(b)
Figure 6. Oxygen concentration curves of Leão coal under air-firing (a) and oxy-fuel (b).
5.2Rates of oxygen molar consumption
3.5e-3
3.5e-3
3.0e-3
3.0e-3
2.5e-3
Rate of oxygen consumption (mol/s)
Rate of oxygen consumption (mol/s)
The rates of oxygen molar consumption for the three investigated coals are compared at 1073 K
(Fig. 7). Lignite presents higher rates of oxygen consumption in both atmospheres. Differences in
ash properties play also an important role in the conversion reactivity. By comparing the two highash content coals, higher reaction rates are observed for the Leão coal because its lower ash content.
However, it is observed a more pronounced influence of oxy-fuel conditions on Bonito coal. This is
explained due to increasing of chemical reaction regime control by the gasification reaction of CO2.
Lignite
Leao
Bonito
2.0e-3
1.5e-3
1.0e-3
5.0e-4
2.5e-3
Lignite
Leao
Bonito
2.0e-3
1.5e-3
1.0e-3
5.0e-4
0.0
0.0
0
5
10
15
20
25
0
5
10
time (s)
15
20
25
time (s)
(a)
(b)
Figure 7. Rate of oxygen consumption under air-firing (a) and oxy-fuel (b) at 1073 K.
5.3 Assessment of integral method of analysis of experimental data
Fig. 8 (a) depicts a representative curve used to fit of the effective reaction rate constant using
the integral method of analysis of experimental data for lignite under O2/CO2 atmosphere at 1073 K.
The effective reaction constant rate was fitted with a reaction order of 0.5 and initial oxygen
concentration of 3.3 mol/m3. The correlation coefficient (R2) obtained with linear least-squares
fitting (LLSF) is 0.99 with a confidence interval of 95%, showing that the applied reaction order
provides an excellent fit to effective reaction rate constant. Nevertheless, it is worthy to mention
that this correlation coefficient is strongly influenced by the reaction rate of the combustion.
4
3.5e-3
Rate of oxygen consumption (mol/s)
3 0.5
Integral Method
keff fitted with reaction order n=0.5
2
2
2
2 (CO ,0(1-XO ))
0.5
(mol/m )
3
1
0
3.0e-3
Mass conservation
Kinetic parameters
2.5e-3
2.0e-3
1.5e-3
1.0e-3
5.0e-4
0.0
0
2
4
6
8
10
12
14
16
18
0
time (s)
2
4
6
8
10
12
14
16
18
time (s)
(a)
(b)
Figure 8. Representative curves showing the fitting of effective reaction rate constant with the integral method (a) and
mass conservation equation (b).
In Fig. 8 (b), the representative curves comparing the rate of oxygen consumption obtained with
reaction rate calculated by the kinetic parameters and with mass conservation equation are
illustrated. The curve of oxygen consumption calculated by the kinetic parameters approximates the
rate of oxygen consumption obtained with mass conservation equation only after 6 s, which
corresponds mainly to char combustion phase. Nevertheless, the results are considered satisfactory
in this case because char conversion is a predominant phase in the whole combustion process.
The behavior of the effective reaction rate constant of lignite along the combustion time under
oxy-fuel conditions at 1073 K with initial oxygen concentration of 3.3 mol/m3 is shown in Fig. 9.
Both curves were obtained by the integral method of analysis of data with reaction order fixed at
0.5, where one represents the values obtained before the integration through Eq. (10), whereas the
other was obtained after the integration process followed by the application of linear regression
LLSF.
This is another approach to interpret the plot in Fig. 8 (a), providing more physical meaning to
the method. It becomes evident the influence of integration on the effective reaction rate constant
along the combustion time. Results after the integration, represented by straight line, are close to the
behavior of reaction rate constant along the time. Through an evaluation of correlation coefficient, it
is possible to confirm the validity of performed fit to the effective reaction rate constant after the
integration, being capable to represent or not the phenomena.
It is important to emphasize that a successful fit also depends on of the combustion reaction
rates. Reactions with higher values such as at 1273 K are difficult to obtain a plateau of effective
reaction rate constant, represented by the curve before integration. It indicates in advance that the
value of correlation coefficient will be far from the unity by LLSF, even for lower reaction orders,
where are usually expected better fittings (Murphy and Shaddix, 2006). This effect can be mitigated
by longer combustion times, as observed in the experimental values obtained at 1073 and 1173 K
with linear least-squares fitting (LLSF).
Effective reaction rate constant
(mol/m ) s
3 0.5 -1
0.8
0.6
keff with n=0.5 before integration
keff with n=0.5 after integration
0.4
0.2
0.0
0
2
4
6
8
10
time (s)
12
14
16
18
Figure 9. Comparison of the effective reaction rate constants before and after the integration.
5.4 Estimation of effective reaction rate constants for evaluation of the fuel reactivity
For the calculation of the effective reaction rate constants of three coals, two main strategies are
adopted in the present study. This is due to the difference of oxygen molar consumption between
the samples, whose oxygen consumption of lignite reaches the double of the values obtained in high
ash content coals. Firstly, the comparisons of the effective reaction rate constants and overall
activation energies are performed on the same basis of the initial oxygen concentration consumption
and same values of reaction orders. In a second approach, due to the lower consumption of oxygen
by the Leão and Bonito samples under air-firing conditions, a comparison with those results
obtained by lignite coal is not performed. Thus, the comparison of the reaction rate constants
between air and oxy-fuel conditions are restricted to the same coal, with exception of Bonito
sample, which does not reach the minimum oxygen consumption to be compared with oxy-fuel
condition.
The plots of oxygen concentration consumption for three coals under oxy-fuel atmosphere at
1073 and 1173 K are shown in Fig. 10. The oxygen molar concentration starts at 2.30 mol/m3. From
the results, it is clear that lignite consumes the same initial oxygen concentration in a noticeable
reduced combustion time at both temperatures. Consequently, higher reaction rates compared to
Leão and Bonito coals are obtained, as observed in Fig. 11. Here, the effective reaction rate
constants under oxy-fuel conditions were fitted with a reaction order of 0.5 and expressed by a
natural logarithm values obtained from linearization of Arrhenius’ equation.
In relation to the high ash content coals, the reaction rates are influenced by the gasification
reaction by CO2 at temperature levels from 1173 K. Conversely, as mentioned previously, the
reaction rate constants of lignite started to be influenced by gasification reaction at lower
temperatures (1073 K). Additionally, it is also observed in this case, that an increase of temperature
levels leads to the combustion to be controlled by both chemical reaction and pore diffusion of
oxygen, according to the Three Zone Theory (Smoot and Smith, 1985).
2.5
3
Oxygen concentration consumption (mol/m )
Oxygen concentration consumption (mol/m3)
2.5
Lignite
Leao
Bonito
2.0
1.5
1.0
0.5
0.0
Lignite
Leao
Bonito
2.0
1.5
1.0
0.5
0.0
0
2
4
6
8
10
12
14
16
18
20
0
2
4
6
8
time (s)
10
12
14
16
18
20
time (s)
(a)
(b)
Figure 10. Oxygen concentration consumption curves under oxy-fuel condition at 1073 (a) and 1173 K (b).
-0.4
Lignite
Leao
Bonito
-0.6
Ln (keff) (mol/m ) s
3 0.5 -1
-0.8
-1.0
-1.2
3
CO2,0=2.30 mol/m
-1.4
-1.6
-1.8
-2.0
7.6e-4
7.8e-4
8.0e-4
8.2e-4
8.4e-4
8.6e-4
8.8e-4
9.0e-4
9.2e-4
1/T (K-1)
9.4e-4
Figure 11. Comparison of the effective reaction rate constants of Lignite, Leão and Bonito coals under oxy-fuel
condition.
A comparison of effective reaction rate constants is presented in Table 4. It is observed that the
correlation coefficient of lignite decreases as the temperature increases due to the influence of
higher reactions rates under diffusion control, reducing the combustion time to consume the same
initial oxygen concentration. For the case of lignite combustion, several reactions orders were
attempted and most suitable fits for constant rates were obtained with values between 0.3 and 0.6.
In order to keep the same conditions to compare the reaction rate constants among the coals, a value
of 0.5 of reaction order was applied in calculations of the kinetic parameters. According to Murphy
and Shaddix (2006), this value is suggested as most appropriate for the char kinetic rate.
Table 4. Comparison of the effective reaction rate constants under oxy-fuel condition. keff (mol/m3)0.5s-1
Temperature Lignite
R2
Time (s)
Leão
Bonito
Lignite
Leão
Bonito
Lignite
Leão
Bonito
1073 K
0.325
0.172
0.158
0.937
0.985
0.990
8.4
16.45
17.25
1173 K
0.460
0.207
0.149
0.902
0.989
0.990
5.5
13.75
18.45
1273 K
0.531
0.453
0.244
0.887
0.946
0.986
4.85
6.15
11.05
In order to show the differences between the effective reaction rate constants calculated under
air and oxy-fuel atmospheres, Fig. 12 depicts the effective reaction rate constants for lignite and
Leão coal. Due to the differences of oxygen consumption between both coals, distinct values of
initial oxygen concentration were adopted for each case in order to compare the effective reaction
rate constants. The initial oxygen concentrations consumed for lignite and Leão coal are 2.7 and 1.7
mol/m3, respectively.
As observed in Fig. 12 (a), the effective reaction rate constants for the German lignite are higher
in oxy-fuel than in air atmospheres for the three levels of combustion temperatures. On the other
hand, significant differences on the reaction rates under oxy-fuel atmospheres for high ash coals are
observed only at temperatures higher than 1173 K (Fig. 12b). As mentioned previously, the
gasification reaction by the higher partial pressure of CO2 is pointed as the main reason in
increasing the reaction rates of coal conversion under oxy-fuel atmospheres. Tables 5 and 6
summarize the values of the effective reaction rate constants as well as correlation coefficients
under air and oxy-fuel conditions for both coals.
-0.4
-0.4
21 vol.% O2 / 79 vol.% CO2
-0.6
Air atmosphere
21 vol.% O2 / 79 vol.% CO2
Air atmosphere
-0.8
3 0.5 -1
-0.8
3
CO2,0=2.7 mol/m
Ln (keff) (mol/m ) s
3 0.5 -1
Ln (keff) (mol/m ) s
-0.6
-1.0
-1.2
3
CO2,0=1.7 mol/m
-1.0
-1.2
-1.4
-1.4
-1.6
-1.6
7.6e-4
7.8e-4
8.0e-4
8.2e-4
8.4e-4
8.6e-4
8.8e-4
9.0e-4
9.2e-4
-1.8
7.6e-4
9.4e-4
7.8e-4
8.0e-4
8.2e-4
1/T (K-1)
8.4e-4
8.6e-4
8.8e-4
9.0e-4
9.2e-4
9.4e-4
1/T (K-1)
(a)
(b)
Figure 12. Comparison of the effective reaction rate constants of lignite (a) and Leão coals for air-firing and oxy-fuel
conditions.
Table 5. Comparison of the effective reaction rate constants of lignite coal between air-firing and oxy-fuel.
3 0.5 -1
R2
keff (mol/m ) s
Time (s)
Temperature
Air
Oxy-fuel
Air
Oxy-fuel
Air
Oxy-fuel
1073 K
0.244
0.297
0.990
0.963
13.25
10.35
1173 K
0.232
0.448
0.963
0.948
14.0
6.55
1273 K
0.302
0.514
0.990
0.922
11.05
5.75
Table 6. Comparison of the effective reaction rate constants of Leão coal between air-firing and oxy-fuel.
3 0.5 -1
R2
keff (mol/m ) s
Time (s)
Temperature
Air
Oxy-fuel
Air
Oxy-fuel
Air
Oxy-fuel
1073 K
0.202
0.220
0.978
0.950
12.25
10.5
1173 K
0.203
0.273
0.980
0.950
12.05
8.55
1273 K
0.315
0.496
0.953
0.892
7.75
4.45
Fig. 13 shows the values of the reaction rate constants for Bonito coal under air atmosphere
conditions, fitted with reaction order of 0.5. Differently from the results obtained for Leão coal, the
reaction rate in the case of Bonito coal, starts taking place at 1073 K. In the first analysis, it is likely
that the Bonito coal is controlled by kinetic regime in lower temperatures, whereas the lignite and
Leão coal are controlled by a diffusion rate regime. The control of the diffusion rate regime in
combustion of lignite coal at similar range of temperature under air atmosphere conditions was also
found in literature (Lorenz and Rau, 1998).
-1.0
Ln (keff) (mol/m ) s
3 0.5 -1
-1.2
Air atmosphere
CO2,0=1.1 mol/m3
-1.4
-1.6
-1.8
-2.0
-2.2
7.6e-4
7.8e-4
8.0e-4
8.2e-4
8.4e-4
8.6e-4
8.8e-4
9.0e-4
9.2e-4
9.4e-4
1/T (K-1)
Figure 13. Effective reaction rate constants of Bonito coal under air-firing.
Table 7 present the values of the reaction rate constants of Bonito coal plotted in Fig.13. The
correlation coefficients and combustion times were determined base on an initial oxygen
concentration of 1.1 mol/m3.
Table 7. Effective reaction rate constants of Bonito coal under air
atmosphere conditions.
Temperature
keff (mol/m3)0.5s-1
R2
Time (s)
1073 K
0.144
0.959
13
1173 K
0.210
0.936
9
1273 K
0.297
0.902
6
5.7 Overall activation energy
Overall activation energy is obtained based on values of reaction rate calculated by Arrhenius’
law equation at three temperature levels. Since linear fitting techniques present a major difficulty
when applied in all range of combustion process, which involve mass transfer and kinetic reactions
rate controlling steps, values of overall activation energy were separated by temperature intervals. It
aims to provide a better understanding on the rate controlling step of the combustion process, being
possible an evaluation by Three Zone Theory proposed by Smoot and Smith (1985).
In Table 8 the values for air-firing combustion are summarized. Lignite and Leão coals present
values of overall activation energy near to zero, since there is no increasing of the effective reaction
rate constants for both coals at temperatures of 1073 and 1173 K, as seen previously in Fig. 12 (a)
and 12 (b). At a temperature range of 1073-1173 K, it seems that the combustion for both coals is
controlled mainly by the mass transfer rate. Chemical reaction rate plays a more important role at
1173-1273 in the case Leão coal, due to the increase of the overall activation energy.
Lorenz and Rau (1998) obtained a set of values of effective reaction rate constants for several
coals utilizing a fluidized bed reactor under air atmosphere conditions at similar temperature range
of the present study. A value 29 kJ/mol with an error of 8 kJ/mol was obtained for lignite in a
fluidized bed reactor. The authors suggest the overall activation energy at a range of 30-40 kJ/mol is
controlled by both chemical reaction rate and mass transfer rate, whereby the latter considerably
prevails. In the present study was encountered a value of the overall activation energy for lignite
under air-firing conditions of 32 kJ/mol, which can be considered similar to previous works.
With respect to the interpretation of combustion behavior of Bonito coal, it seems that the
combustion process is more influenced by chemical reaction than the diffusion rate control at first
and second intervals of temperature, since there is an increasing of the effective reaction rate
constants with the increasing of temperature, as seen in Fig. 13.
Table 8. Overall activation energies under air-firing condition. Coal
E (kJ/mol)
1073 – 1173
K
Lignite
Leão
Bonito
0
0
39.5
1173 – 1273
K
Lignite
Leão
Bonito
32
54
43
Temperature
interval
Values of overall activation energies under oxy-fuel atmosphere conditions are presented in
Table 9. It is observed at first interval of temperature (1073-1173 K) that overall activation energy
of lignite is controlled both by the chemical kinetic and diffusion rates, prevailing the latter at
second interval of temperature (1173-1273 K), due to the decrease of the overall activation energy.
Conversely, both Leão and Bonito coals have their overall activation energies increased under oxyfuel atmosphere conditions at second interval of temperature. Several authors mention, that values
of 90 kJ/mol indicate that chemical reaction is responsible for controlling the rates of combustion
(Lorenz and Rau, 1998; Murphy and Shaddix, 2006; Milioli, 2008, Cuesta et al., 1993). It could be
considerably affected in such cases by the gasification reaction due to higher concentration of CO2.
Table 9. Overall activation energies under oxy-fuel condition.
Temperature
interval
Coal
E (kJ/mol)
1073 – 1173
K
Lignite
Leão
Bonito
36
19
0
1173 – 1273
K
Lignite
Leão
Bonito
18
97
60
6.CONCLUSIONS
This work was aimed at a fundamental study of the kinetics of combustion of one pre-dried
lignite and two-high ash content coals under air and oxyfuel conditions. The reactor utilized in the
experimental work is a laboratory-size atmospheric cyclone combustion chamber. The overall
combustion kinetics was followed by the measurements of O2 concentration, temperature and flow
rates. Preliminary combustion experiments were performed aiming to determine suitable values of
overall kinetic data, as well as to investigate the influence of higher partial pressure of CO2 on the
conversion of coal under oxy-fuel conditions. The strategy adopted for the calculation of the global
kinetic parameters applied the mass of oxygen consumed during the combustion of a sample.
Higher effective reaction rate constants were obtained under oxy-fuel condition for lignite and
Leão coal in comparison with air-firing. Influence of gasification reaction by the CO2, which
overcomes the effects of low diffusivity of oxygen in higher CO2 partial pressures, were observed.
Comparison of global kinetic parameters of Bonito coal obtained under oxy-fuel with air
atmosphere conditions was not feasible because of the low oxygen consumption found. Further tests
will be performed with samples greater than 1 g in order to compare the global kinetic parameters
of Bonito and Leão coals with lignite under air atmosphere conditions.
As expected, lignite showed highest effective reaction rate constants under oxy-fuel condition.
This behavior is related to the high content of ash in Leão and Bonito coals, which reduces the
chemical reaction rate control at lower temperatures (1073 – 1173 K), prevailing the diffusion rate
regime. On the other hand, at a higher temperature interval (1173 – 1273 K), Leão and Bonito coals
showed an increasing of the chemical reaction rate control over the diffusion regime. Additionally,
values of overall activation energy were higher than those found under air atmosphere, which can
be explained by the influence of the gasification reaction of CO2. Since the control of the
combustion reaction in low rank coals with high content of ash by chemical reaction and mass
transfer rates is much more complex with higher CO2 partial pressures, further tests are still
necessary to understand the overall phenomena.
With respect to the linearization method applied to obtain the effective reaction rate constants, it
is worthy to emphasize that higher reaction rates strongly influence the linear fitting, reducing the
correlation coefficient value and penalizing the integral method of analysis to obtain the kinetic
parameters. Based on these preliminary conclusions, nonlinear regression techniques are planned to
be implemented in order to achieve a better fitting of effective reaction rate constants.
7.ACKNOWLEDGEMENTS
The authors gratefully acknowledge the financial support received for this research from Federal
Ministry of Education and Research (BMBF) through the Innoprofile Research Program, German
Academic Exchange Service (DAAD) and the National Council of Technological and Scientific
Department (CNPq)
8.REFERENCES
Cuesta A., Martínez-Alonso, A., Tascón, J.M.D. Correlation between Arrhenius Kinetic Parameters
in the Reaction of Different Carbon Materials with Oxygen. Energy & Fuels 1993; 7; 1141-1145.
Levenspiel, O. Chemical Reaction Engineering, 3rd Edition, 1999, John Wiley & Sons, New York,
USA.
Lorenz, H., Rau, H. A new method for investigating the combustion behavior of solid fuel in FBC.
Fuel 1998; 77; 127-134.
Montgomery, D.C. Design and Analysis of Experiments, 5th Edition, 2001, John Wiley & Sons,
New York, USA.
Murphy, J.J, Shaddix, C.R. Combustion kinetics of coals chars in oxygen-enriched environments.
Combustion and Flame 2006;144; 710-729.
Olajire AA. CO2 capture and separation technologies for end-of-pipe applications - A review.
Energy 2010;35:2610-28.
Rathnam, R.K., Elliot, L.K., Wall, T., Liu, Y., Moghtaderi, B., 2009. Differences in reactivity of
pulverized coal in air (O2/N2) and oxy-fuel (O2/CO2) conditions. Fuel Processing Technology
2009;90; 797-802.
Stanmore B.R., Visona S.P. The Contribution to char burnout from gasification by H2O and CO2
during pulverized-coal flame combustion. Combustion and Flame 1998; 113; 274-276.
Tappe, S., Krautz, H.J. ALVA 20: A 20 kWth Atmospheric Laboratory Test Facility to Investigate
the Combustion Behaviour under Close-to-Reality Conditions. Proceedings of the European
Combustion Meeting 2009;06.
Toftegaard MB, Brix J, Jensen PA, Glarborg P, Jensen AD. Oxy-fuel combustion of solid fuels.
Progress in Energy and Combustion Science 2010;36:581-625.
Turns, S.R. An introduction to combustion: concepts and applications. McGraw Hill International
Editions, 2nd Edition, 2006, printed in Singapore.
Wall, T.; Combustion Processes for Carbon Capture. Proceedings of the Combustion Institute 2007;
31: 31-47.
Zhang, L., Binner, E., Chen, L., Qiao, Y., Li, C., Bhattacharya, S., Ninomiya, Y. Experimental
Investigation of the Combustion of Bituminous Coal in Air and O2/CO2 Mixtures: 1. Particle
Imaging of the Combustion of Coal and Char. Energy Fuels 2010; 24; 4803–4811
9. NOMENCLATURE
C
Molar concentration, mol/m³
E
Overall activation energy, kJ/mol
f
Molar fraction, -
keff
Effective reaction rate constant, (mol m-3)n-1 s-1
ko
Pre-exponential factor, (mol m-3)n-1 s-1
N
Number of moles, -
n
Order of reaction, -
n
Molar flow, mol/s
Patm Atmospheric pressure, atm
R
Constant of ideal gas, 8.314 J/(mol K)
R2 Correlation coefficient, r
Reaction rate, (mol m-3 s-1)
T
Temperature, K
t
Time, s
V
Volume of reactor, m³
V
Volumetric flow rate, m³/s
X
Conversion, -
Abbreviations
CCS Carbon dioxide capture and storage
CFD
Computational fluid dynamics
daf
Dry, ash free
LLSF Linear least-squares fitting
Subscripts
0
Initial
atm Atmospheric
cons Consumed
eff Effective
in
Inlet
out Outlet
tot Total