27/03/2015
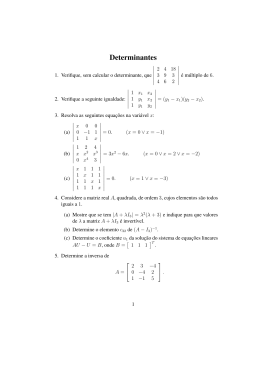
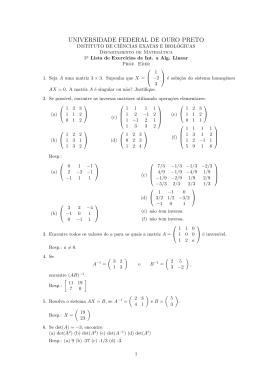
2a Lista de GAAL-COMPUTAÇÃO, LICENCIATURA
Matriz Inversa e Sistemas
1. Determine a matriz inversa da matriz:
[
A=
12 7
5 3
]



−2 −1 0
2
1 −1 1
 3
1 −2 −2



; B =  2 −1 3  ; C = 
 −4 −1 2
3
0 1 3
3
1 −1 −2







;D = 




1
2
3
4
5
0
1
2
3
4
0
0
1
2
3
0
0
0
1
2
0
0
0
0
1




.



2. Verificar quais das seguintes matrizes são invertı́veis e deterinar as inversas suas inversas.




0
0
1
1
[
]
1 0 1
 1 0 0 1 
1 2




A=
;B =  1 1 0 ;C = 
.
 1 1 1 −1 
2 2
0 2 1
0 2 0 3
3. Para cada um dos sistemas a seguir, interprete cada equação como uma reta no plano, faça
gráfico dessas retas e determine geometricamente o número de soluções.

{
{
{

=
 x1 + x2
x1 + x2 = 4
x1 + 2x2
= 4
2x1 − x2
= 3
(a)
(b)
(c)
(d)
x1 − x2
=

x1 − x2 = 2
−2x1 − 4x2 = 4
−4x1 + 2x2 = −6

−x1 + 3x2 =
4.
 Use o método da substituição para resolver o sistema abaixo.
x1 + 2x2 + 2x3 + x4 = 5




3x2 + x3 − 2x4 = 1

−x3 + 2x4 = −1



4x4 = 4
5. Escreva cada um dos sistemas
a seguir como

{

x+y
=

2x + 2y = 1
(a)
(b)
2x + y − z =

2x − 3y = 5

3x − 2y + 2z =
6. Resolver os seguintes
 sistemas de
{

 x + 2y + z
x−y = 4
i.
ii.
y + 2z

x+y = 0

x+y+z

x−y+z+t = 0



 x+y−z+t = 1
iv.

−x + y + z − t = 0



2x − y − z + 3t = 1
uma equação
matricial.


5
 2x + y + z = 4
6 (c)
x − y + 2z = 2


3x − 2y − z = 0
7
Cramer:


= 1
 x+y+z = 2
= −4 iii.
x−y+z = 0


= 2
y + 2z
= 0
7.
 Determinar m ∈ IR de modo que o sistema abaixo seja de Cramer e, a seguir, resolvê-lo:

 x − y + 2z = 2
x + 2z
= 1


x + 2y + mz = 0
o
1
1
3