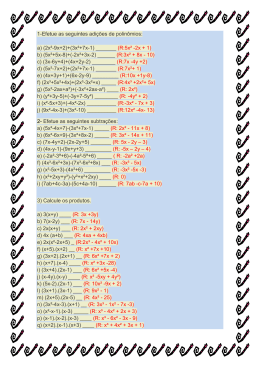
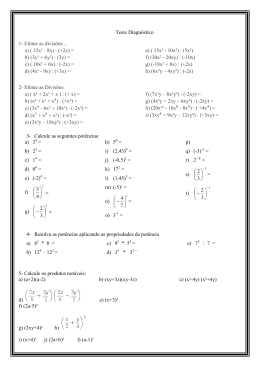
Lista 17 de CDI 1
EDOL de ordem superior: Equações Homogêneas
1. Defina se as funções são linearmente independentes ou dependentes no intervalo (−∞, +∞).
1.1) f (x) = x,
f (x) = x2 ,
1.2) f (x) = 5,
f (x) = cos2 x,
f (x) = sen2 x
f (x) = 1,
f (x) = cos2 x
1.3) f (x) = cos 2x,
1.4) f (x) = x,
f (x) = x − 1,
1.5) f (x) = 1 + x,
1.6) f (x) = ex ,
f (x) = 4x − 3x2
f (x) = x + 3
f (x) = x2
f (x) = x,
f (x) = e−x ,
f (x) = senh x
2. Verifique se as funções dadas formam um conjunto fundamental de soluções para a equação diferencial,
no intervalo indicado, e construa a solução geral.
2.1) y 00 − y 0 − 12y = 0,
e−3x ,
2.2) y 00 − 2y 0 + 5y = 0,
ex cos 2x,
2.3) 4y 00 − 4y 0 + y = 0,
ex/2 ,
2.4) x2 y 00 − 6xy 0 + 12y = 0,
2.5) y (4) + y 00 = 0,
1,
(−∞, +∞)
ex sen 2x,
xex/2 ,
x3 ,
x,
e4x ,
x4 ,
cos x,
(−∞, +∞)
(−∞, +∞)
(0, +∞)
sen x,
(−∞, +∞)
3. Encontre uma segunda solução para cada equação diferencial e suponha um intervalo apropriado.
3.1) y 00 − y 0 = 0;
3.3) y 00 − 25y = 0;
3.5) xy 00 + y 0 = 0;
y1 = 1
y1 = e5x
y1 = ln x
3.2) y 00 + 9y = 0;
3.4) x2 y 00 + 2xy 0 − 6y = 0;
3.6) (1 − x2 )y 00 − 2xy 0 = 0;
y1 = sen 3x
y 1 = x2
y1 = 1
1
3.7) (1 + 2x)y 00 + 4xy 0 − 4y = 0;
3.9) x2 y 00 − 4xy 0 + 6y = 0;
y1 = e−2x
y 1 = x2 + x3
3.8) x2 y 00 − 20y = 0;
3.10) xy 00 − (x + 1)y 0 + y = 0;
y1 = x−4
y1 = ex
4. Encontre a solução para cada equação diferencial.
4.1) 2y 00 − 5y 0 = 0
4.5) 8y 00 + 2y 0 − 1y = 0
4.9) y 000 + y 00 − 4y = 0
4.2) y 00 − 8y = 0
4.6) 2y 00 − 3y 0 + 4y = 0
4.10) y 000 + 3y 00 + 3y 0 + y = 0
4.3) y 00 − 3y 0 + 2y = 0
4.7) 4y 000 + 4y 00 + y 0 = 0
4.4) y 00 + 3y 0 − 5y = 0
4.8) y 000 + 3y 00 − 4y 0 − 12y = 0
5. Resolva a equação diferencial sujeita às condições iniciais indicadas.
5.1) y 00 + 16y = 0;
5.5) y 00 + y = 0;
y(0) = 2, y 0 (0) = −2
y(π/3) = 0, y 0 (π/3) = 2
5.2) y 00 + 6y 0 + 5y = 0;
5.6) y 000 + 2y 00 − 5y 0 − 6y = 0;
y(0) = 0, y 0 (0) = 3
y(0) = 0, y 0 (0) = 0, y 00 (0) = 1
5.3) 2y 00 − 2y 0 + y = 0;
5.7) y 000 − 8y = 0;
y(0) = −1, y 0 (0) = 0
y(0) = 0, y 0 (0) = −1, y 00 (0) = 0
5.4) y 00 + y 0 + 2y = 0;
5.8) y iv − y = 0;
y(0) = 0, y 0 (0) = 0
y(0) = 0, y 0 (0) = 0, y 00 (0) = 0, y 000 (0) = 1
2